Strike and dip is a convention for measuring the attitude of a planar structure, such as a bedding plane, fault, joint or vein, in terms of the angles which it makes with the geographic coordinates of the earth’s surface – north-south, east-west and up-down. You probably know all this – it is pretty much geology 101 as the Americans say – but bear with me, I intend to start with some basic definitions and work my way up to ideas that may not be so familiar to you.
The strike of the surface to be measured is defined as the orientation of a horizontal line[1] on the surface using the 000°-360° divisions of the compass. Thus, if the horizontal line trends exactly north-east its strike is 045°. Exactly south is a strike of 180°; exactly NW a strike of 215° and so on. Since a line has two ends, its strike could be expressed by either of two numbers – the 045° strike of our first example could also be quoted as its reciprocal 225°; a 180° strike as 000° (or 360°), the 215° strike as 135° etc.. Unless you are using the Right-Hand-Rule (which I explain below), there is no general rule for which number you quote for a strike measurement: it is optional.
The dip of the surface being measured is the acute angle which the surface makes with the horizontal. The measurement is made downwards from the horizontal in the vertical plane which is at right angles to the strike. Dips are measured from 00° (that is: horizontal) to 90° (vertical). More strictly, this measurement is known as the True Dip. If the dip measurement is taken in any plane other than the plane at right angles to strike, the figure obtained is called an Apparent Dip. Apparent dips are always less than true dips. In some circumstances you may not be sure whether your dip reading is apparent or true, but you can be sure that the true dip is at least as great as the figure you have obtained.
When recording strike and dip, if three digits are always used for the strike (eg 000, 045, 135, 354) and a double digit used for the dip (00-90) the two measurements can always be distinguished. As an aside: don’t ever quote a strike as being so many degrees west or east of north. This is sloppy and unscientific. Compass directions are always measured and quoted as degrees clockwise from north. That’s it. No other comment or annotation is necessary.
To fully define and understand the attitude of a planar surface encountered in outcrop or drill core a geologist needs to know its strike, its dip and the direction of the dip towards one of the principal quadrants of the compass. When making a geological map, the strike is usually the most important measurement, because it is that which defines the potential continuity of the surface in the horizontal plane.
A geological map is a graphical way of showing the distribution of strike. A section is a graphical way of showing the distribution of dip.
When measurements are recorded digitally (as opposed to analog recording as a strike and dip symbol on a map) the most common traditional way has been in the form of xxx / yy / A, where xxx (the strike) is a 3-digit compass bearing (000°- 360°), yy (the dip) a two digit number representing the angle from the horizontal (00°- 90°) and A is the direction of dip towards a principal compass direction or quadrant (i.e. N, NE, E, SE, S, SW, W or NW). As an example: 042/23 NW is a surface with strike of 042° that dips at 23° to the northwest. Because this method requires three data fields (strike, dip and dip direction) the advent of computer-based databases has lead to the use of dip and dip direction, utilising only two data fields. The dip measurement is exactly as before: the dip direction is a compass bearing exactly 090° different (either plus or minus) to the strike. It is expressed as yy/zzz or yy to zzz . It is simple mental arithmetic to derive strike/dip/dip direction from dip/dip direction, or vice versa.
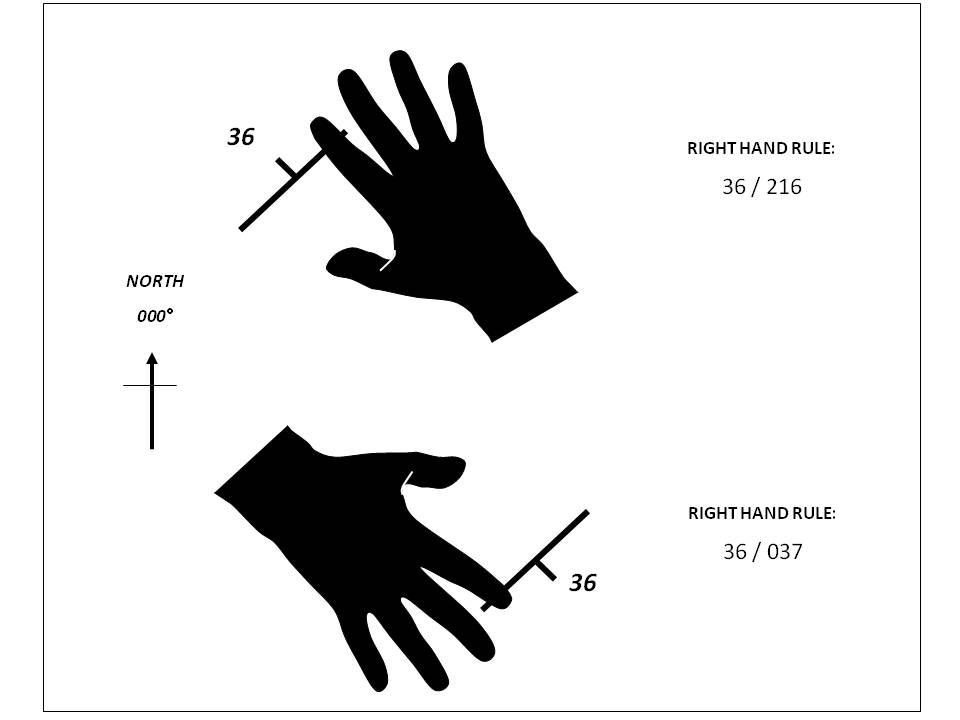 The Right Hand Rule for recording dip and strike measurements
The Right Hand Rule for recording dip and strike measurements
A third way of quoting the attitude of a plane is employed by some geologists. In this method, the plane is measured and recorded digitally simply as a strike and dip. The all-important dip direction qualifier is then recorded by means of a convention in the way the strike number is expressed. The most common of these conventions is the so-called “Right-Hand Rule” (see figure above). This rule can be explained thus: imagine grasping a strike/dip map symbol with the right hand, palm down and fingers pointing in the direction of dip. The thumb then indicates the strike direction to be recorded. For example: an east-west strike (090°-270°) with a 60° dip to the north would be recorded as 270/60. A record of 090/60 would indicate the same strike but a dip of 60° to the south. The right-hand rule is an excellent system, but has one disadvantage - a near fatal one in my opinion. Since it is not universally employed, you can never be sure when looking at someone’s map, table or report that it has been employed, unless the geologist specifically and prominently draws attention to its use. In the absence of such information, you might be wrong to assume that an attitude quoted as yy/xxx involves the RHR (some other convention might be employed or else the geologist simply forgot the dip qualifier).
Comparison of three measuring conventions for the attitude of planes
Strike/dip/dip direction Right Hand Rule Dip/dip direction
xxx/yy/A xxx/yy xx to zzz
037/36 SE 037/36 36 to 127
217/36 SE 037/36 36 to 137
037/36 NW 217/36 36 to 307
The attitude of linear structure is measured and recorded as its trend and plunge Trend is defined as the horizontal direction or strike of a vertical plane passing through the lineation, measured in the direction of plunge. It is recorded as a compass bearing between 000° and 360°. Plunge is the angle that the lineation makes with the horizontal, measured in the vertical plane. A measurement of 76/067 represents a plunge of 76° to 067°. If a lineation lies in a plane, then it can be measured as its pitch on that plane. A pitch is the angle that a lineation makes with the horizontal, measured in the plane that contains the lineation. If the attitude of the plane is also known, then knowing the pitch enables the trend and plunge to be calculated. The simplest way to do this is by means of a stereonet.
Block diagram illustrating the strike and dip of a plane, the trend and plunge of a lineation on a plane and the pitch of a lineation on a plane.
Any computer software used should be capable of accepting and presenting data in all the above formats.
[1] any horizontal line – if the surface is planar, they are all parallel
